Circles
K-12: Materials at high school level.
Some Examples
A circle is a set of points that all lie at the same distance from a fixed point, called center. Circles come in all different sizes. We sometimes measure the distance from the center to the point on the circle and this distance is called the radius.
Circles show up in everyday life and below are some examples. In the top row we start of with the circle we just described. It has center C, radius r and a point P on the circle. Next to it is a roud sign for a round about. this is a place where the rooad forms a circle and in the sign the circle is made up of three curved arrows. Another example of circular shapes is a coin. Here we show a quarter, but if you check any loose change you - or your friend - may have in his/her pocket you will see that all our coins are circular in shape.
In architecture and design circular chapes can be used. The example shown on the bottom row is a circular patio with a fountain in the center. If you have ever done any do-it-yourself work you will recognize the circular saw shown on the bottom row. Aas the name says, the saw is shaped as a circle. Finally on the bottom right we have included a print from MC Escher. It is called Circle Limit III and is one out of a series of 4 prints.
 |
 |

|
| Circle with center C and radius r | Road sign: circular intersection | A Quarter |
 |
 |

|
| Circular Patio | Circular Saw | Escher's Circle Limit III |
There are many more examples of circular shapes. Think about your CDs, DVDs, dinnerplates, etc. Circles really are everywhere!
Drawing Circles
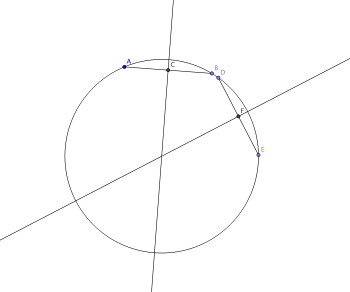
We can draw circles in different ways and in different combinations. We can try to draw circles free hand, or we can use a compass. The following diagram shows how to draw circles with a compass. You bend the legs of the compass so that the distance between the tip of the pencil and the sharp point is the same size as the radius you want. Put the point of the compass at the location of the center of the circle and rotate the compass around to draw the circle.
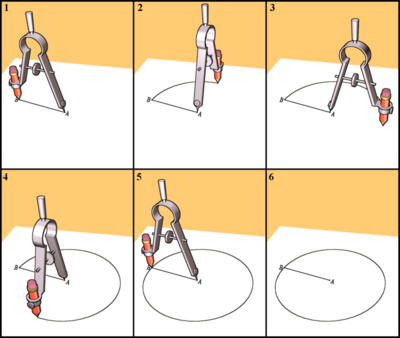
Below we see several circles drawn with the same center (A). If the circles have the same center and different radius, then they are called concentric. We can also draw several circles with different centers and different radius.
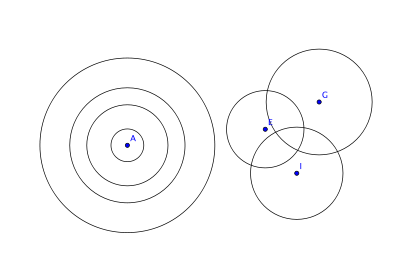
We can ask several types of questions about the circle. One may be that we want to draw a circle to have one shape inside and the other outside the circle. We have done an example for you and you can see the pictures that go with it below.
What if a center is given with a triangle T and a polygon P1, and we want the circle big enough so that P1 lies inside the circle and T lies outside the circle? In this case that can be done and the circle is shown in the picture on the right.
 |

|
| Triangle T, Polygon P1 and the center | The circle drawn in so that T lies outside and P1 inside the circle |
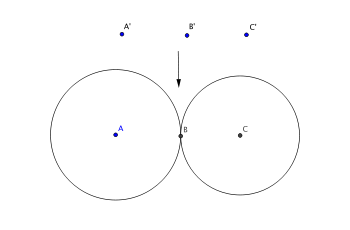
Sometimes you will be asked to draw a circle of a specific radius. Suppose you are given 3 points as shown at the top of the picture here. The points are labeled A, B, and C. We want a circle with center A and radius AB and we want another circle with radius C and radius BC. We can use our compass to draw those circles.
Radius, Diameter and Chord
The radius is the length of the segment that connects the center to any point on the circle. The diameter is the distance across the circle. The diameter is always twice the size of the radius.
A chord is just any line segment between two points on the circle. In the picture below segment AB is the radius of the circle. Segment CD is the diameter. Note that the diameter always passes through the center of the circle. Segment FH is a chord.
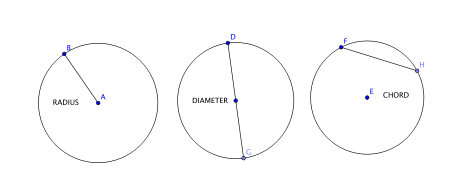
The radius and the diameter tell us how big the circle is. We use the radius for instance if we want to compute the circumference of the circle (this measures how long the actual circular curve is), and we use the radius when we want to find out how much area the circle takes up.
If we have 2 chords, we can actually find out where the center of the circle is. Suppose for instance someone gives you a circle, but they forgot to mark the center. This means that we cannot find the radius of the circle or the diameter. If we needed to do some computations for the circle this would be a problem.
Below you see a circle. We drew 2 chords: AB and DE. Next we constructed a line that cut the chord in half and was perpendicular (at 90 degrees) to the chord. We did this for both chords.
Notice that the two new lines intersect in a point. This intersection point is the center of the circle!