Copy of a previous Final

Final Math 124 Spring 2010 Name:______________________

PART I – Multiple Choice (40 points total)

Give the best – and most complete – answer

1. When determining the symmetry groups for border patterns
 A. There are 3 possible symmetry groups
 B. There are 7 possible symmetry groups
 C. There are 17 possible symmetry groups
 D. There are infinitely many possible symmetry groups

2. When determining the symmetry groups for rozette patterns
 A. There are 3 possible symmetry groups
 B. There are 7 possible symmetry groups
 C. There are 17 possible symmetry groups
 D. There are infinitely many possible symmetry groups

3. When determining the symmetry groups for wallpaper patterns
 A. There are 3 possible symmetry groups
 B. There are 7 possible symmetry groups
 C. There are 17 possible symmetry groups
 D. There are infinitely many possible symmetry groups

4. In border patterns we can have:
 A. Only 2 fold rotational symmetry
 B. Only 2-, 3-, 4- and/or 6-fold rotational symmetry
 C. Any degree rotational symmetry
 D. No rotational symmetry at all

5. In wallpaper patterns we can have:
 A. Only 2 fold rotational symmetry
 B. Only 2-, 3-, 4- and/or 6-fold rotational symmetry
 C. Any degree rotational symmetry
 D. No rotational symmetry at all

6. In Spherical geometry the sum of the angles in a triangles
 A. Has to be exactly 180 degrees
 B. Has to be strictly less than 180 degrees
 C. Has to be strictly greater than 180 degrees
 D. Could be anything.

7. In hyperbolic geometry the sum of the angles in a triangles
 A. Has to be exactly 180 degrees
 B. Has to be strictly less than 180 degrees
 C. Has to be strictly greater than 180 degrees
 D. Could be anything.

8. Escher created works in
 A. Euclidean and spherical geometry only.
 B. Euclidean and hyperbolic geometry only
 C. Euclidean, spherical and hyperbolic geometry
 D. Four different geometries.

9. The fifth axiom for Spherical geometry says that
 A. Given a line and a point not on the line there is only one parallel line
 B. There are no parallel lines.
 C. There are many parallel lines but they look very different
 D. Only antipodal points have parallel lines.

10. A regular 4-gon is a 4-sided figure with congruent angles and congruent sides.
 A. Regular 4-gons exist in Euclidean geometry
 B. Regular 4-gons exist in spherical geometry
 C. Regular 4-gons exist in hyperbolic geometry
 D. All of the above

PART II - Symmetry Groups (65 points total)

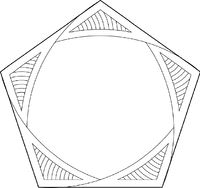
1. (10 pts) Answer the following questions:

Reflections

Mark all the mirror lines in this image

Mark the center of rotation C in the image above.

The rotation is ______ fold

This is a rotation of __________degrees

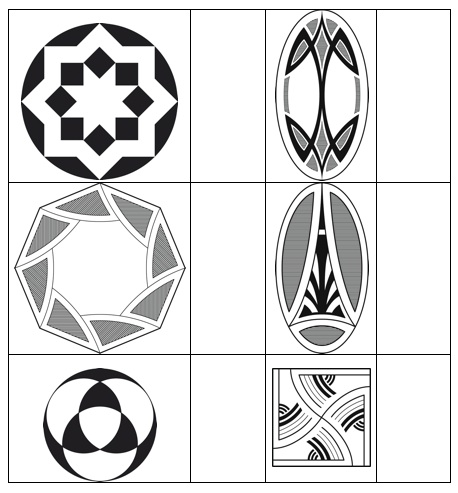
2. (15 pts) Give the symmetry group (i.e Cn or Dn, with appropriate value of n) for the following rosettes. Write the group in the space to the right of the rosette. No explanations needed.

3. (20 pts) Border Patterns: Answer questions (and follow directions) given below each image.

Make sure you mark the translation (simple arrow)

True False This border pattern has 2-fold rotations. (Cirle) Also mark all of them on the image if they exist.

True False There are vertical mirror lines.

True False There are horizontal mirror lines.

The border pattern has symmetry group __________________

Make sure you mark the translation (simple arrow)

True False This border pattern has 2-fold rotations. (Cirle) Also mark all of them on the image if they exist.

True False There are vertical mirror lines.

True False There are horizontal mirror lines.

The border pattern has symmetry group __________________

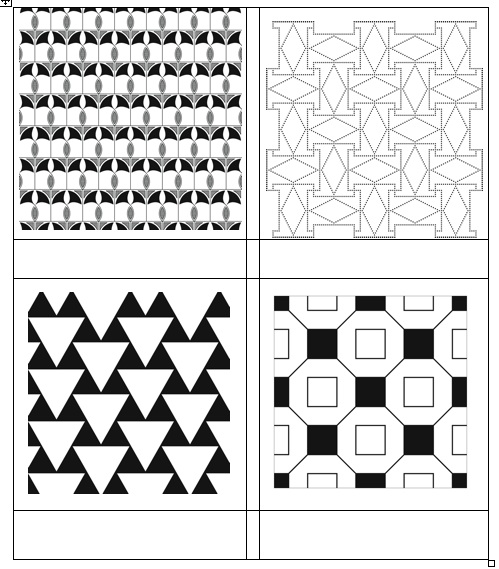
4. (20 pts) Find the wallpaper groups of the following 4 patterns. If there are mirror lines in the tessellation, then draw in all visible ones.

PART III– Theory of Tessellations (20 points total)

1. (15 pts) We can prove that all triangles tessellate. Below is an outline of the proof and you will be asked to justify some of the steps. We will assume that a parallelogram is defined to be a 4 sided polygon, whose opposite angles are congruent. A. Draw an arbitrary triangle (so any one you like). Assume it has angles x, y and z. Rotate the triangle about the midpoint of one of its sides (180 degrees). Draw the picture, and explain why the resulting figure is a parallelogram.

B. Explain why parallelograms tessellate the plane. Why do they cover the plane without gaps or overlaps?

C Why does this show that all triangles tessellate? Why do they cover the plane without gaps or overlaps? (Look at parts A and B.)

2. (5 pts) Regular 5-gons do not tessellate. Using these shapes would always result in either a gap or an overlap. Explain why this is the case. (Hint: look at the angle measure.)

PART IV– Spherical and hyperbolic Geometry (25 points total)

1. (10 pts) a. Draw a 90-90-90 degree triangle on a sphere:

b. Draw 2 different bi-angles on the sphere:

2. (5 pts) a. Draw a 90-5-5 degree triangle in the hyperbolic disk:

3. (10 pts) Draw a tessellation by ideal 4-gons. Decorate it using a method of your choice:

NOTE TO SPRING 2011 students: We did not pay much attention to this topic this semester. Ignore this question.

PART IV– Impossible figures (10 points total)

1. We looked at impossible figures. Choose one of the following Escher works and describe why they are impossible.

On the next 2 pages you see larger images. Feel free to mark them up, draw diagrams on them, and in general explain as much as you can about these images. [I did not add larger images in this online version]