Instructor:Polygon Exercises Solutions

Polygon Exercises

1. The examples should be varied. See the text for examples of rectangles, parallelograms and trapezoids. Examples of kites can include the following:
2. The rectangles are a subclass of the class of parallelograms. In other words: Every rectangle is a parallelogram.
3. Sketch of argument: Suppose the angles are labeled X and Y.

   Then 2X + 2Y = 360. And hence X + Y = 180. This means that the bottom of the figures forms a straight line. Note that the vertical segments meet the horizontal lines and that alternate interior angles are congruent. This implies that the horizontal lines must be parallel to one another. A similar argument shows that the sides must be parallel as well ad that the quadrilateral must be a parallellogram.

4. a. T b. T c. T d. T e. F f. T
5. There are 3 lines of symmetry in an equilateral triangle.
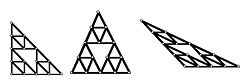
6. Within each triangle all drawn triangles are similar. Congruence requires the triangles to also have the same size. This means that in each of the examples there is 1 large triangle, there are 4 congruent medium triangles, and there are 12 congruent small triangles.
7. The examples constructed depend on the side chosen. Technically all the resulting shapes are parallelograms (although some of the special cases can be rectangles or a rhombus). For example: rotated right triangles will give a rectangle or a paralellogram (possibly a square if it's an isosceles rigth triangle). 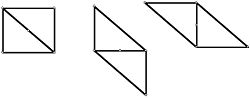By carefully keeping track of the angles while rotating, we see that the quadrilaterals always have the property that opposite angles are equal. By problem 3 we see that this means that the resulting quadrilateral is always a parallelogram.
8. All triangles tessellate. Check that the triangles are really isosceles (part a) and scalene (part b). The tessellation needs to be large enough to establish a pattern.
9. All triangles tessellate the plane. Students may or may not have noticed this at this stage. Check that they give some reasoning. Make sure they use full sentences hen answering questions.
10. All quadrilaterals tessellate. Like #8 and 9 this is going to motivate what we do later. Students may not be completely correct. Working the problem is all that counts at this stage.
11. All quadrilaterals tessellate. Students may or may not have noticed this at this stage. Check that they give some reasoning. Make sure they use full sentences hen answering questions.
12. Check that they show enough of the tessellation to show a pattern.
13. All three of the cubist paintings show a variety of geometric shapes. How they are used and the complexity of the shapes is rather different however. Braque is the simplest. He uses rectangles, triangles and (convex) quadrilaterals. Picasso uses mainly triangles and fairly simple quadrilaterals, but they tend to overlap more. Metzinger uses triangles, trapezoids, rectangles, etc. But note that he also uses a non-convex quadrilateral in the foreground (and another in the background) and in the back are examples of (half hidden) 5- and 7-gons!