Introduction to Non-Euclidean Geometry
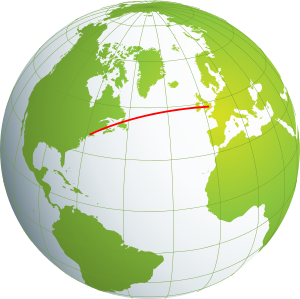
So far we have looked at what is commonly called Euclidean geometry. There are occasions where this type of geometry doesn’t get one very far. Suppose we look at a globe model of the Earth and want to measure the distance between New York and London. A ruler won't work, because the ruler will not lie flat on the sphere to measure the length. And if measuring length on the Earth is already tricky, how would you find area, even of a relatively simple shape like the state of Colorado?
Geometry was initially developed to measure things: length, area and volume. Geometric measurements were used in ancient Egyptian and Babylonian times to measure the level of the Nile, to build temples, to construct the pyramids, and to measure land for taxation. The basic objects in geometry are lines, line segments, circles and angles. These four objects are the topic of the first axioms of geometry, sometimes called postulates. The fifth postulate in traditional Euclidean geometry describes parallel lines, and will prove to be the most interesting.
What are the key features of lines and line segments? One important answer is that they measure the shortest distance between two points. Builders use this property to lay out straight lines at a construction site. They put two stakes in the ground and pull a piece of string taut between them.
To measure the distance between two points on a sphere one might could do the same thing: put two stakes on the sphere and pull a piece of string taut between them. This procedure produces curves called geodesics, which are curves that minimize the distance between their endpoints. On a spherical surface such as the Earth, geodesics are segments of curves called great circles. On a globe, the equator and longitude lines are examples of great circles.
Non-Euclidean geometry is the study of geometry on surfaces which are not flat. Because the surface is curved, there are no straight lines in the traditional sense, but these distance minimizing curves known as geodesics will play the role of straight lines in these new geometries. Then the geodesics are used as the basic object to create non-euclidean circles, triangles and other polygons.

On the right, consider the sphere with three geodesics (solid great circles). One is the equator, and the other two cross the equator and each other at 90° angles. These great circles are faintly visible as they continue all the way around the back of the sphere, where they meet again.
The three intersections of these geodesics which are visible on the front of the sphere define three geodesic segments that form a spherical triangle. Spherical triangles can behave in very strange ways. This is a 90°-90°-90° equilateral triangle - a triangle with three right angles. Such a triangle only exists on the sphere! There is no way to draw a 90°-90°-90° triangle on a piece of flat paper paper. The sum of the angles of a Euclidean triangle is always 180, and this picture shows a spherical triangle whose angles sum to 270°.
Another dramatic difference between Euclidean and non-Euclidean geometry is with parallel lines. Two lines are parallel if they never meet, and much of high school geometry class involves playing with properties of parallel lines. However, on a sphere any two great circles will intersect in two points. This means that it is not possible to draw parallel lines on a sphere, which also eliminates all parallelograms and even squares and rectangles.
In developing non-Euclidean geometry, we will rely heavily on our knowledge of Euclidean geometry for ideas, methods, and intuition. However, major differences do appear and it is important to pay special attention to the 'rules' for Euclidean geometry that these new geometries will shatter.
Explorations and reading assignments
The following reading assignment will help students critically read the section:
Famous Early Geometers
- Pythagoras (ca. 540 BC) Showed that in a right triangle the sum of the squares of the sides equals the square of the hypotenuse.
- Plato (ca 380 BC) Laid the basis for formal geometry. His name is associated with the Platonic solids. Above the entrance to his school of Philosophy (the Academy) was engraved : “Let no one ignorant of geometry enter my doors”
- Aristotle (ca 340 BC) The tutor of Alexander the Great, also trained many of the great geometers of the time.
- Euclid (ca 300 BC) The first to write down the postulates for what is now known as Euclidean geometry. He was associated with the famous School of Alexandria.
- Archimedes (ca 225 BC) Pliny called him “the God of Mathematics”. He was also associated with the School of Alexandria. His name is now associated with the Archimedean solids. He was killed during the Siege of Syracuse. He was so immersed in his math that he supposedly did not notice the city being taken over by the Romans.
- Eratosthenes (276-194 BC) Realized that the earth was round, and was able to compute a fair approximation of its circumference.
- Plutarch : “God eternally geometrizes”
The geometry taught in elementary, middle, and high-school is all Euclidean geometry. As you can see above, many of the results we learn about have been known for over 2000 years. Mathematicians have investigated properties of spherical triangles for almost 2000 years, but no systematic theory for geometries other than Euclidean geometry were developed until the 18th and 19th centuries. Contributors include Lambert, Saccheri, Lobachevsky, Bolyai, Gauss, and Riemann. At the end of the 19th century it was recognized that there were different systems of geometry. We will investigate spherical geometry (the geometry of the sphere) and hyperbolic geometry (as illustrated by Escher’s prints Circle Limit I-IV).