Spherical Geometry: Isometry Exploration

Objective:

Extend concepts of reflection, rotation and translation to the sphere

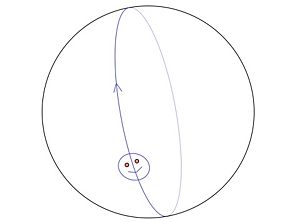
1. On the sphere below sketch several images of the smiley face under translation. Can we translate the image to the back of the sphere? Why or why not? What happens if our translation distance is greater than the circumference of the circle?

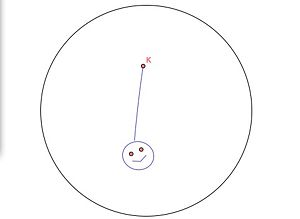
2. Rotate the smiley face through an angle of 45 degrees about K in a counter clockwise direction.

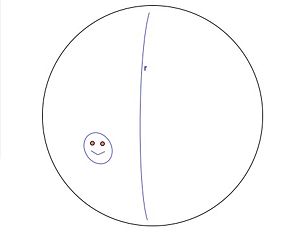
3. Reflect the smiley face across line segment r.

4. Create a glide-reflection using line r as the taxis of the glide-reflection (i.e., using it to indicate direction and for reflection).

5. • How are translations on the sphere different from those in the plane? How are they similar?
   • How are rotations on the sphere different from those in the plane? How are they similar?
   • How are reflections on the sphere different from those in the plane? How are they similar?
   • How are glide-reflections on the sphere different from those in the plane? How are they similar?
6. • In the plane, translations are compositions of reflections. Draw a sketch showing how two reflections across parallel lines result in a translation. (Illustrate it with a set of points or an asymmetric figure fairly close to one of the reflection lines.)
   • What about on the sphere--does the same thing work?
7. • In the plane, rotations are compositions of reflections. Draw a sketch showing how two reflections across intersecting lines result in a rotation. (Illustrate it with a set of points or an asymmetric figure fairly close to one of the reflection lines.) What is the center of the resulting rotation?
   • What about on the sphere--does the same thing work?
8. In the plane rotations and translations are different. On the sphere however these two isometries are really the same.
   • Consider a translation on the sphere along some geodesic. To argue that this is a rotation we would have to find the center of rotation. What is the center of rotation? Draw a picture showing that a translation really can be realized as a rotation.
   • Now consider a rotation on the sphere. This can also be seen as a translation. What is the geodesic along which we are translating?

Handin: A sheet with answers to all questions.