The Axioms of Spherical Geometry Exploration
Objective: Understand the axioms of spherical geometry.
The axioms of Euclidean geometry give us the basic statements from which the rest of Euclidean geometry can be deduced. They basically describe the behavior of lines, segments, circles, angles and parallel lines.
Below write down the respective axioms for spherical geometry. Illustrate with example(s0 when appropriate.
Remember that the role of lines is played by the great circles - also known as geodesics - in spherical geometry.
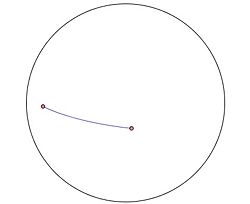
Lines
Euclidean Geometry: Any two points can be joined by a straight line. (This line is unique given that the points are distinct).
Spherical Geometry:
Case 1: Points A and B are not antipodal.

Case 2: Points A and B are antipodal.

Segments
Euclidean Geometry: Any straight line segment can be extended indefinitely in a straight line.
Circles
Euclidean Geometry: Given any straight line segment, a circle can be drawn having the segment as radius and one endpoint as center.
Spherical Geometry:
Angles
Euclidean Geometry: All right angles are congruent.
Spherical Geometry:
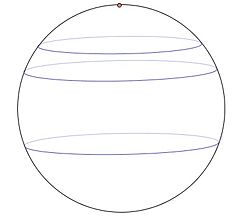
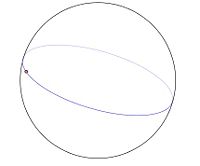
Parallel Lines
Euclidean Geometry: Through a point not on a given straight line, one and only one line can be drawn that never meets the given line.
Are the following curves examples of spherical parallel lines? Explain why or why not.
Handin: A copy of this sheet with answers to the questions.