The Geometry of Antoni Gaudi
Antoni Gaudi i Cornet (1852-1926) was a well-known architect from Spain. He was born in 1852 as the son of a copper-smith. He studied architecture in Barcelona and combined an interest in history, mathematics and nature to create a rather unique style.
Fairly early in his career Gaudi made a display case for a glove maker from Barcelona, which was on display during the world fair in Paris in 1889. The wealthy entrepeneur Eusebi Güell i Bacigalupi noticed the display case, and upon his return to Barcelona he sought out Gaudi. This was the start of a lifelong friendship and collaboration. Güell had Gaudi design his main residence Palau Güell as well as Parc Güell in Barcelona. One of Gaudi's greatest works however became the Temple of the Holy Family (the Sagrada Familia) in Barcelona. [1]
Magic Square
The passion facade of the Sagrada Familia was also worked on by the Catalan sculptor Josep Maria Subirachs (1927 -).
Subirachs created a magic square where the rows and columns add up to 33, the age of Jesus at the time of his death. Two numbers are repeated: 10 and 14. If we add these numbers we get 48. The number 48 is the same number one obtains if the letter of INRI (Iesus Nazarenus Rex Iudeourum) are assigned a number according to their order in the Latin Alphabet.
| A | B | C | D | E | F | G | H | I | K | L | M | N | O | P | Q | R | S | T | V | X |
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 |
So that 9 + 13 + 17 + 9 = 48
Tesselations
Gaudi used mosaics in many of his works and he created several tiled floors and ceilings in the houses and parks he designed. The mosaics used in Gaudi's work are an example of Catalan modernism and are sometimes referred to as trencadís.
The pillars in the Greek Theatre (Teatre Greque) show a random tessellation. The mosaic used to cover the surface of the pillar is laid out with no discernible pattern. The wall at the Parc Guell shows an overall pattern made up out of squares, but the colored mosaic squares all have their own (sometimes) symmetric pattern.
There are several true periodic tessellations. Many of them are based on the square, but there are also a couple of tessellations based on the hexagon and a wood inlay with a pattern consisting of triangles.
 |
 |
|
| A tessellation based on squares. | Another tessellation based on squares, | A hexagonal tessellation, but only 3-fold symmetry. |
 |
 |
|
| Tessellation and optical illusion | Triangular tessellation | Another tiling |
Catenary Arches and Catenoids
A catenary arch is the shape one gets when we suspend a rope or chain from its endpoints. Gaudi used catenary arches in many of his projects. The advantage of the catenary arch is that it can be constructed from relatively light materials while still being able to support great weights.
In La Pedrera (also known as Casa Milà) a model of suspended chains is on view. A mirror below the model shows the reflected image of the structures. The reflected image clearly shows a collection of arched buildings formed by the catenoids, In the Sagrada Familia a similar model is on display, but the chains are now weighted with small bags. The latter model corresponds to the church that was planned at Santa Coloma de Cervello. The church was however never completed and at the present only the crypt is completed.
 |
|
| Suspended chains form catenoids | The reflection shows at outline of arched buildings |
 |
|
| Model with weights | The design of the Church at Santa Coloma de Cervello. |
Conic Sections
Parabolas
The parabola is a curve created when we slice a double cone at an angle. There are other definitions of the parabola, but the definitions of the parabola as a conic section is the easiest to visualize. Gaudi used the parabolic arch to great effect. One of the first times he used the parabolic arch was in his design of the Palau Guell in Barcelona. The entrance gates are in the shape of parabolic arches. [1]
 |
 |
|
| Parabolic cross section of a cone. | Parabolic entryway to Palau Guell | The attic in Casa Batllo. |
Hyperbolas
The hyperbola is a curve created when we slice a double cone vertically. This type of curve is used in the interior of the Sagrada Familia. The curves create fairly high vaults, and in the main church Gaudi used this to create pillars that resemble the structure of of tree complete with branched tree trunks.
 |
 |
|
| Cross section of a double cone. | Sketch of a cross section showing hyperbolas | Ceiling of the Sagrada Familia. |
Below are examples of several cross sections of the cone, showing the hyperbolic, parabolic and elliptic cross sections. On the right, a close up of the ceiling is shown with a hyperbolic curve superimposed to show where the hyperbolic curve is located.
 |
|
| Cross section of a cone. | Close-up showing hyperbolas |
Ruled Surfaces
Ruled surfaces are created by sweeping a line through space.[2] A simple example of a ruled surface is the cylinder one gets if we connect all the points in one circle with their corresponding point on another circle (see image below in the hyperboloid of one sheet section). Gaudi used several of these ruled surfaces in his designs.
Hyperboloids of One Sheet
A hyperboloid can be created if a column of strings is twisted about its central axis. Gaudi used this type of curved surface in the construction of some of the windows in the Sagrada Família in Barcelona.
The cloister walls have window created from 10 hyperboloid sheets which are arranged in a hexagonal honeycomb pattern.
 |
 |
|
| Twisting a cylinder gives a hyperboloid. | Model of Hyperboloid | Cloister wall, Sagrada Familia. |
Hyperbolic Paraboloids
The hyperbolic paraboloid looks somewhat like a saddle. A simple formula for such a surface is z = x y. [3]
 |
 |
|
| Model of hyperbolic paraboloid from the Museum at the Sagrada Familia | Computer generated model | Arch by Gaudi |
Some of the cross sections of the hyperbolic paraboloids are parabolas. This can be used to create parabolic arches. The image on the right shows a saddle surface and the surface connects to pillars.
Fun Fact: Pringles potato chips are hyperbolic paraboloids!
Which arch is it?
There are parabolic arches, hyperbolic arches and catenary arches. How do we tell them apart? That can be quite tricky actually. There are no obvious visual clues, so that the identification usually comes from the notes by the architect. It had been noted by Galileo Galilei (in 1638) that a suspended chain almost forms a parabola, but that a parabola is slightly less curved. Galileo had also observed that if the angle of the arch was less then 45 degrees the catenary and the parabola "agree almost to within a hair". [4] That the curve now known as a catenary is not a parabola was shown in the late 17th century, and the real equation for the catenary was derived in 1691 by such famous scientists as Leibniz, Huygens and Johann Bernouilli. [5]
The importance of catenary arches in the construction of buildings has been attributed to Robert Hooke (ca 1671). [6] He has been quoted as saying: "As hangs a flexible cable so, inverted, stand the touching pieces of an arch."
Below you will see several examples of these arches and it should become clear just how similar they look. It is very interesting then that they do have very different properties when it comes to architecture. And it does make a difference which one is used in the construction of arches! This shows the importance of mathematics in art and architecture.
 |
 |
|
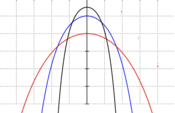
| Parabola | Hyperbolic arches | Catenary arches |
Other
This example of a ruled surface shows nicely how it is created by sweeping out a straight line. This is a model for the roof of the school building associated with the Sagrada Familia.[1]
St Louis Connections
There are several interesting architectural sites in St Louis (home town of the author).
- The St Louis Gateway Arch is (roughly) a catenary arch. The arch was designed by architect Eero Saarinen and structural engineer Hannskarl Bandel in 1947.
- The McDonnell Planetarium at the St. Louis Science Center is a hyperboloid of one sheet.
- The Millennium Hotel in downtown St. Louis is a cylinder, and as such a a ruled surface.
References
- ↑ 1.0 1.1 1.2 Rainer Zerbst, Antoni Gaudi: Al zijn bouwwerken ("Antoni Gaudi: All of his stuctures"), 2012 (orig. 1985), Taschen GmbH
- ↑ Weisstein, Eric W. "Ruled Surface." From MathWorld--A Wolfram Web Resource. http://mathworld.wolfram.com/RuledSurface.html
- ↑ Weisstein, Eric W. "Hyperbolic Paraboloid." From MathWorld--A Wolfram Web Resource. http://mathworld.wolfram.com/HyperbolicParaboloid.html
- ↑ Fahie, John Joseph (1903). Galileo, His Life and Work. J. Murray. pp. 359–360. Google Books
- ↑ Lockwood, E.H. (1961). "Chapter 13: The Tractrix and Catenary". A Book of Curves. Cambridge.
- ↑ Lisa Jardine, Monuments and Microscopes: Scientific Thinking on a Grand Scale in the Early Royal Society, Notes and Records of the Royal Society of London, Vol. 55, No. 2 (May, 2001), pp. 289-308, JSTOR access
