The Mathematical Papyri

Introduction

Some historical background

The Rhind mathematical papyrus

The first sources about ancient Egyptian mathematics date to what is called the Middle Kingdom. The Egyptian civilization rose and declined several times. The Old Kingdom represents the first rise of the Egyptian civilization. During this period the country was unified and the great pyramids are constructed. We do not have a lot of information about this period besides the main monuments of the rulers and the higher ranking officials. We do not have any scientific writing from this period. As a result egyptologists are still not sure for instance how the pyramids were constructed.

The Egytian state went into a decline after the time of the great pyramid builders and the civilization rose to greatness again several centuries later. From this time period we have scientific writings. The Moscow papyrus dates to roughly 1700 BCE and contains several algebraic and geometric problems together with their solutions. Some of the geometry problems concern the volume of a truncated pyramid, the surface area of a hemispherical bowl to give some examples. Several papyri from this time period were found in Lahun (sometimes called Illahun) and nearby Kahun. Some of these texts concern the bookkeeping of royal estates and some include mathematical writings.

One of the biggest resources we have for mathematical writing is the Rhind papyrus, named after Alexander Rhind who purchased the papyrus in the 19th century and brought it to England. The papyrus is sometimes called the Ahmes papyrus after the scribe who wrote it. This papyrus contains an inscription dating it to the time of a pharaoh named Khamudy. This must be one of the smaller rulers from the northern part of Egypt from ca. 1650 BCE. The country was in decline at that point, and this period is referred to as the second intermediate period.

There are other sources of mathematics from the long history of Ancient Egypt. On this page a selection of some of the more famous writings concerning geometry have been included.

The mathematics as known from these sources

It should be noted that we only have a handful of resources that show us what mathematical knowledge the Egyptians had. It is possible more was known but that the writings were lost over the course of time.

From the limited information we have it appears that the Egyptians did not develop much in the form of theory. There is not much facility with symbolic manipulation that can be gathered from the texts either. The mathematics seems to have been experimentally based. They were able to develop methods for finding solutions to common problems. The papyri consist of lists of problems with their solutions. Occasionally a method for checking ones work is given, so that the scribe could check their work was correct. If this is truly the state of Egyptian mathematics of that time (and not a misconception due to the limited information we have), then this paints a picture of people who quite ingeniously were able to develop all the necessary mathematical tools and techniques to construct all their great monuments and administer their estates without having the benefits of theoretical mathematics.

The papyri contain many types of mathematical problems. A famous fact about Egyptian mathematics is that they used unit fractions. They used 1/2, 1/3, 1/4, 1/5, etc. The only non-unit fraction they used was 2/3. This means that their computations could at times be somewhat laborious. Note for instance that in the Rhind papyrus problem 56, the answer is given as "1/2 + 1/5 + 1/50". We would have brought the numbers over a common denominator and expressed that answer as 36/50. To deal with fraction, the Egyptians used tables. Some of these tables have been preserved in the mathematical papyri. A nice description can be found at [mathpages]. It is not known why the Egyptians wrote their fractions like this.

Egyptian terms used in the papyri

The peremus is the height of an object. In hieroglyphics it looks like this:

The ukha-thebet is the lenght of an object. In hieroglyphics it looks like this:

The seqed measures the slope of an angle. In hieroglyphics it looks like this:

In the literature we also find the spelling seked. The seqed can be thought of - in modern terms - as computing the cotangent of an angle. It computes the ratio run/rise.

The Egyptians used several different units of measurement. A larger list can be found in Rossi (page 61). Some units of measurement used in the examples below are:

Length:

• The (royal) cubit (meh in Egyptian) was approximately 52.5 cm (= 20.7 in. = 1' 8.7"). The royal cubit was a principal unit of measurement in architecture. The egyptians also used what was called the "small cubit". The small cubit was approximately the length of a forearm (45 cm = 17.7 in). The royal cubit was equal to 7 palms.
• The palm (shesep in Egyptian) was approximately 7.5 cm (= 3 in) long. The measurement - and the name - seem to have been derived from the actual width of a person's palm. One palm was equal to 4 fingers.
• The finger (djeba in Egyptian) was one of the smallest units of length and measured ca. 1.875 cm (= .7 in). This is approximately the actual width of a finger.
• The khet, also called the "rod of cord" may have referred to a knotted cord 100 cubits long. A khet measured ca. 52.5 m (= 172' 2.9").

Area:

• The setat is 1 square khet, which is 10,000 square cubits.

Volume and weight:

• The hekat is equivalent to ca. 4.8 liters.
• The deben is a measure of weight. In the early history of Ancient Egypt is was equivalent to 13.6 grammes. After ca. 1600 BCE is was equivalent to 91 grammes.

Egyptian Geometry

We only have a limited number of problems from ancient Egypt that concern geometry. Geometric problems show that they knew how to compute areas of several geometric shapes and volumes of cylinders and pyramids.

Computing Triangles

It is clear that they knew that the area of a triangle is 1/2*base*height. Problem 51 in RMP and problem 7 in MMP use this formula.

Problem 51 RMP Example of producing (i.e. Calculating) [the area] of a triangle (spdt) of land. What is the area of a triangle of height 10 khet and a base of 4 khet? The answer is computed to be 20 setjat. (1/2*4*10).

Problem 4 of the MMP is a copy of problem 51 of the RMP.

Problem 7 MMP. "A triangle of given area is such that it altitude is 2.5 times its base; find both." . More specifically the problem states: “[There is a triangle with area 20 [setjat] and 'bank' (idb – ratio of height to base) of 2 ½; find the length and the breadth of the triangle.

Problem 17 MMP. Given a triangle with area 20 (setjat) and a length that is 1/3+1/5 of its breadth; Find the length and breadth. (The answer is length 10 and breadth 4)

Computing Rectangles

Problem 49 RMP. Find the area of a rectangular plot of land measuring 10 khet by 2. (There may be an error in the papyrus here; it possibly should be a 10 by 1 area?)

Problem 6 MMP. Given a rectangular enclosure of 12 units area and the ratio of the sides as 1 : 3/4, find the lengths of the sides. This problem seems to be identical to one of the Kahun Papyri in London. The problem is also interesting because it is clear that the Egyptians were familiar with square roots. They even had a special hieroglyph for finding a square root. It looks like a corner and appears in the fifth line of the problem. We suspect that they had tables giving the square roots of some often used numbers. No such tables have been found however.

Problem 6 from the Moscow Mathematical Papyrus

[line 1] Example of Calculating a rectangle [line 2] If someone says to you: A rectangle is 12 setjat [in area] [has a breadth 1/2 1/4 [i.e. 3/4] of its length.[Calculate it] [line 3] Calculate 1/2 1/4 to get 1. The result is 1 1/3 [line 4] Take this 12 setjat 1 1/3 times. The result is 16. [line 5] Calculate its square root. The result is 4 for its length [and] 1/2 1/4 of it is 3 for the breadth

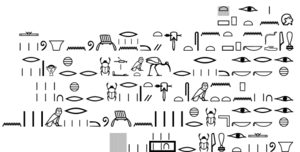
At the bottom (sixth line) is an illustration showing a rectangle with area 12 written in the interior, 4 at the top (in Clagett's book it's 3 at the top, bt that clearly should be a 4) and 3 at its side.

The steps of the solution given in both papyri is the same:

$1:3/4=4/3;\ 4/3*12=16;\ {\sqrt {16}}=4\ (=x);\ 4*3/4=3\ (=y)$

Problem 18 MMP. Find the area of a length of garment-cloth measuring 5 cubits 5 pals by 2 palms. (The answer is 80 [square palms])

Lahun Papyrus Problem 1 in LV.4 is given as: "An area of 40 "mH" by 3 "mH" shall be divided in 10 areas, each of which shall have a width that is 1/2 1/4 of their length." A translation of the problem and its solution as it appears on the fragment is given on the website maintained by University College London.

Circles and Areas of More Complicated Forms

Problem 48 RMP Compare the area of a circle (approximated by an octagon) and its circumscribing square. This problem's result is used in problem 50.

Trisect each side. Remove the corner triangles. The resulting octagonal figure approximates the circle. The area of the octagonal figure is:

$9^{2}-4{\frac {1}{2}}(3)(3)=63$

Next we approximate 63 to be 64 and note that $64=8^{2}$

Thus the number $4({\frac {8}{9}})^{2}=3{\frac {13}{81}}$ plays the role of π.

That this octagonal figure, whose area is easily calculated, so accurately approximates the area of the circle is just plain good luck.

Obtaining a better approximation to the area using finer divisions of a square and a similar argument is not simple.

Problem 50 RMP. Find the area of a round field of diameter 9 khet. This is solved by using the approximation that circular field of diameter 9 has the same area as a square of side 8.

Problem 52 RMP Find the area of a trapezium with (apparently) equally slanting sides. The lengths of the parallel sides and the distance between them being the given numbers.

Problem 10 MMP. Computes the area of a hemisphere.

Volumes of Cylinders

Problem 41 RMP. Computes the volume of a cylindrical granary of diameter 9 and height 10.

Problem 42 RMP. Computes the volume of a cylindrical granary of diameter 8 and height 6. (or possibly height 9 and breadth 6? There seem to be different translations of this problem.)

Problem 43 RMP. Computes the volume of a cylindrical granary of diameter 9 and height 10.

Problem 60 RMP. This exercise seems to concern a pillar or a cone instead of a pyramid. It is rather small and steep, with a seked of four palms (per cubit). The problem and its solution as decribed in the papyrus are given in Clagett.

[In] a pillar (iwn) [or perhaps a cone?] with a base side (sntt) [or perhaps diameter] of 15 cubits and a height of 30 [ubits]; what is its seqed?

Take 1/2 of 15; it is 7 1/2. Operate on 30 so as to get 7 1/2. The result is 1/4; which is the seqed.

Lahun Papyrus A problem appearing in IV.3 computes the volume of a granary with a circular base of diameter 12 "mH" and height 8 "mH". A similar problem and procedure can be found in the Rhind papyrus (problem 43).

Volumes of Rectangular Parallellopipeds

Problen 44 RMP. Computes the volume of a rectangular granary of length 10, breadth 10 and height 10.

Problems 45 and 46 RMP Discuss the volumes and dimensions of Parallelopipedal granaries.

Problem 14 MMP. The base is a square of side 4 cubits, the top is a square of side 2 cubits and the height of the truncated pyramid is 6 cubits.

Last part of problem 14 from the Moscow Mathematical Papyrus. The real inscription is in hieratic; this is the hieroglyphic transcription of the text. The glyphs start at: You are to add the 16 and the 8 and the 4 - See below.

The text reads:

" Example of calculating a truncated pyramid. If you are told: a truncated pyramid of 6 for the vertical height by 4 on the base by 2 on top:

You are to square this 4; result 16. You are to double 4; result 8.

You are to square this 2; result 4. You are to add the 16 and the 8 and the 4; result 28.

You are to take 1/3 of 6; result 2. You are to take 28 twice; result 56. See it is of 56.

You will find (it) right."

Pyramids

Problem 56 RMP This problem indicates an understanding of the idea of geometric similarity. This problem discusses the ratio run/rise, also known as the seqed. Such a formula would be need for building pyramids.

In modern terms the problem essentially asks to compute the cotangent for some angle. Note that the Egyptians never used any angle measures, so they technically did not use any trigonometry as these formulas would not have held any meaning for them.

Example of reckoning a pyramid 360 in its "ukha-thebet" (lenght of the base) and 250 in its "peremus" (height).

Cause thou that I know the seked of it. You are to take half of 360; It becomes 180. You are to reckon with 250 to find 180. Result: 1/2 + 1/5 + 1/50.

A cubit being 7 palms, you are to multiply by 7.

1 ---- 7

1/2 --- 3 + 1/2

1/5 --- 1 + 1/3 + 1/15

1/50 --- 1/10 + 1/25

Its seked is 5 1/25 palms.

Problem 57 RMP. The height of a pyramid is calculated from the base length and the seked (egyptian for slope).

A pyramid 140 in its ukha-thebt (length of the base), and 5 palms, 1 finger in its seked. What is the peremus thereof?

You are to divide 1 cubit by the seked doubled, which amounts to 10 1/2.

You are to reckon with 10 1/2 to find 7 for this one cubit.

Reckon with 10 1/2.

Two-thirds of 10 1/2 is 7.

You are to reckon with 140, for this is the ukha thebt.

Make two-thirds of 140, namely 93 1/3.

This is the peremus thereof.

Problem 58 RMP. Determine the seked, given the length of the base and the height. The seked is again five palms, one finger (per cubit). In Clagett the exact problem is given as:

In a pyramid whose altitude is 93 1/3 make known the seqed of it when its base side is 140 [cubits]." (The answer is 5 palms 1 finger)

Problem 59 RMP. This exercise contains two parts.

59A In a pyramid whose base side is 12 [cubits] and whose altitude is 8 [cubits]; what is its seqed? (Answer: 5 palms 1 finger)

59B may be a computation to check the answer: If you construct a pyramid with base side 12 [cubits] and with a seqed of 5 palms 1 finger; what is its altitude? (Answer: 8 cubits)

Mathematical sources

The Rhind Mathematical Papyrus

This papyrus is sometimes called the Ahmes papyrys. Written by a scribe named Ahmes (Ahmose) in ca. 1550 BCE and bought in modern times by Alexander Henry Rhind. The papyrus lists many problems covering topics in algebra, geometry and other areas in mathematics.

In the Rhind papyrus there are nineteen problems of a geometric nature: nos. 41-46 and 48-60. The first six problems show how to calculate volume. The problems include finding volumes of right circular cylinders (granaries) and finding volumes and dimensions of paralellopipedal granaries.

Several problems from nos. 48-60 show how to compute area. Formulas are given for the area of a triangle and several four sided figures. The remaining problems "deal with the relation of the lengths of two sides of a right triangle which corresponds to the cotangent of e angle which a face of a regular pyramid makes with its base. This is called the seked of the pyramid."

The Moscow Papyrus

The Moscow mathematical papyrus

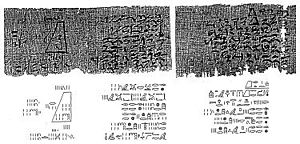
The Moscow Mathematical Papyrus is also called the Golenischev Mathematical Papyrus, after its first owner, Egyptologist Vladimir Goleniščev. The Papyrus dates to the Middle Kingdom, ca. 1700 BCE. The Moscow papyrus is smaller than the Rhind papyrus. The most often quoted problem is problem 14. In this problem the volume of a truncated pyramid is computed.

Berlin Papyrus

Also known as Berlin P. 6619, this papyrus dates to the Middle Kingdom. It dates to roughly the same historical period as the Moscow papyrus mentioned above. The papyrus contains two problems that solve simultaneous equations. Another papyrus now in Berlin (P. 11529) contains exercises that compute the volume of a tapered column. P. 11529 dates to the second century AD and appears to contain several mistakes.

Egyptian Mathematical Leather Roll

The leather roll was bought in Egypt by Rhind at approximately the same time that the Rhind Mathematical Papyrus was purchased (mid 19th century). The problems are mainly algebraic in nature. "It consists of 26 unit fraction series each of which is an expression of a rational number of the form or into an Egyptian fraction.".

Lahun Papyri

The Lahun papyri consist of several fragments. They are sometime referred to as the Kahun papyri. The papyri date to the Middle Kingdom which is the same time period that gave us the Moscow papyrus. The papyri were discovered in the late 19th centuy in the city of Lahun in Egypt.

A problem appearing in IV.3 computes the volume of a granary with a circular base of diameter 12 "mH" and height 8 "mH". A similar problem and procedure can be found in the Rhind papyrus (problem 43).

Problem 1 in LV.4 is given as: "An area of 40 "mH" by 3 "mH" shall be divided in 10 areas, each of which shall have a width that is 1/2 1/4 of their length."

A translation of the problem and its solution as it appears on the fragment is given on the website maintained by University College London.

The other mathematical problems in these papyrus fragments are algebraic in nature.

Explorations

• Egyptian Geometry Eploration
• The Seqed and Modern Trigonometry Exploration