The Pyramids

Introduction

The Step pyramid of Djoser at Saqqara. Ca 2575 BCE (Photo: Jon Bodsworth)

The pyramids in Egypt developed in several stages. The first pyramid dates back to the third dynasty. King Djoser had a step pyramid constructed at Saqqara. Djoser's chief architect Imhotep is credited with developing the step pyramid from the older mastaba tomb. The mastaba is thought by some to represent a bed. Mastabas are large rectangular structures that form the monument for the deceased. The dead were buried in shafts that were sunk into the ground. Imhotep stacked successively smaller rectangular shaped structures to create what is now known as the step pyramid.

Miroslav Verner . writes: "At the outset the structure had the form of a square mastaba (stage M1), which was gradually enlarged, first equally on all four sides (stage M2) and then only o the east side (stage M2). The mastaba <...> already had a step shape in the M3 stage. The step-shaped mastaba was finally built in two stages, first as a four step (P1) and then as a six-step (P2) pyramid, which no longer had - and this is a noteworthy point - a square base; it now had a rectangular base, oriented east-west."

The collapsed pyramid at Meidum. Time of Pharaoh Sneferu (Photo: Jon Bodsworth)

Approximately fifty years after the reign of Djoser, Egypt was ruled by Pharaoh Sneferu. During the reign of this king the first true (smooth faced) pyramids were developed. The Egyptians seem to have used some trial and error methods to develop the appropriate angles of the faces. There are two rather interesting pyramids dating to the time of Sneferu that give us some insight into the developmental stages of the pyramid construction.

The bent pyramid of Sneferu at Dashur. ca. 2575 BCE (Photo: Jon Bodsworth)

A pyramid in Meidum collapsed at some point. It has been suggested that it collapsed while under construction. But recent excavations seem to show that the structure slowly lost its cohesion.

In nearby Dashur Snefru's bent pyramid was started at a rather steep angle of incline measuring 60 degrees, but several adjustments had to be made. The subsurface at Dashur was likely to soft to support the downward forces of a pyramid built at a steep inclination. Originally the angle of inclination was adjusted down from 60 degrees to not quite 55. This required the Egyptian builders to enlarge the base. But halfway through the construction the angle was again changed. The bottom part of the structure was left as it was, but the rest of the pyramid had an angle of only 45 degrees. The latter adjustment gives the pyramid it's peculiar bent shape.

The Pyramids at Giza

The Pyramids at Giza

The three pyramids at Giza - just outside Cairo - are the pyramids most people will first think of when they hear the word pyramid. Of the three, the Great Pyramid or the Pyramid of Khufu (known as Cheops to the Greeks), is the one people talk about the most. Khufu was the son of Senfru mentioned above and he moved the royal necropolis to Giza. He started a truly ambitious building program there. The great pyramid is just one part of a large complex. The Great Pyramid has three smaller pyramids standing next to it for Queens, there is a staellite or cult pyramid, five boatpits were constructed and ancient boats were buried near the pyramid. All of this was accompanied by two temples and a large causeway. For the royal family tombs were constructed nearby in the form of so called mastabas ("beds").

The great pyramid has a square base measuring 230.4 meters, and a height of 146.5 meters. Note that this gives a seqed of approximately .78. This is very close to the seqed of a 3-4-5 triangle where we take the base to be 3 (see below).

The other two pyramids, belonging to Khufu's son Khafre (Chefren in Greek) and grand-son Menkaure (Mycerinos in Greek) are ever so slightly smaller and do not receive the same attention as the great pyramid of Khufu in popular literature.

Pyramids, the Pythagorean Theorem and Special Triangles

One theory about the construction of the pyramids involves the use of special right triangles. The Egyptians may have used (wooden?)right triangles during the construction process to help determine the slope of the pyramid.

Illustration of the Pythagorean Theorem. Note that $a^{2}+b^{2}=c^{2}$

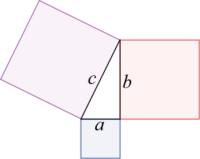
The Pythagorean Theorem states that given a right triangle with sides of length a and b respectively and a hypothenuse of length c, the lengths satisfy the equation $a^{2}+b^{2}=c^{2}$. There are some special cases where the lengths are integers. One very famous example is the 3-4-5 triangle.

A 3-4-5 triangle

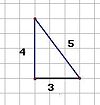
For the triangle depicted here for instance the seqed would be the run / the rise, which in this case is 3/4 = .75 The angle made between the base and the hypothenuse is $53.13^{o}$. Imagine a triangle like this being used while constructing the layers of a pyramid, before the sides are smoothed out. Then by creating a rise of say 4 feet for every horizontal change of 3 feet, would give a general outline of a shape with an angle of about $53.13^{o}$. This is actually extremely close to the angle of incline of the second pyramid at Giza - the pyramid of King Khafre - which has an angle of incline measuring $53.1^{o}$. This does not prove beyond a shadow of a doubt that the pyramid builders used right triangles to establish the slopes, but it is an interesting possibility.

The Right Triangle and the Pyramids Exploration will explore the connection between right triangles and the pyramids further.

Pyramids and Mathematical Papyri

Problems 56 - 59 of the Rhind Mathematical Papyrus compute the seqed of a triangle. The seqed is related to the slope of the side of the pyramid. If we look at a triangular cross section, then the seqed is defined to be (1/2 base of the pyramid) / height.