Circle Limit Exploration

Objective: Explore Escher's Circle Limit prints to develop an intuition for hyperbolic geometry

Materials

• Printed copies of Circle Limit I, Circle Limit II, Circle Limit III, and Circle Limit IV (Heaven and Hell).

Circle Limit I

Recall that in Spherical Geometry, the shortest path between two points is along a great circle. These shortest paths are called geodesics, and the geodesics play the same role as do straight lines in Euclidean geometry. Escher's Circle Limit prints are based on a new kind of geometry, Hyperbolic Geometry.

M.C. Escher, Circle Limit I (1958) with geodesics in red.

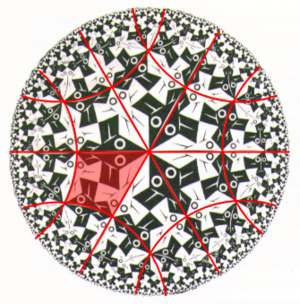
The red lines shown on Circle Limit I are geodesics in this new geometry. These curves will play the role of straight lines. Each red line follows the spines of a line of fish.

1. There are two types of red line marked in the Circle Limit I figure. Describe them. Draw more geodesics by following the spines of other rows of fish. Describe the curves that result.

In these pictures of hyperbolic geometry, geodesics come in two forms, either straight lines through the center of the disk, or arcs of circles that meet the disk's edge at 90°. Segments of geodesics form the sides of polygons. Polygons in hypebolic geometry will look "pinched" to our Euclidean eyes.

2. What type of polygons do you see in Circle Limit I?
3. Compare the angle sum of one of these polygons to the corresponding angle sum for Euclidean geometry.

Circle Limit I is a picture of a surface called "hyperbolic space", but it is a distorted picture. In actual hyperbolic space, these fish would all have the same size and shape.

4. What is the highest order of rotation symmetry for this print?
5. Describe the geometric tessellation underlying Circle Limit I.

Circle Limits II and IV

M.C. Escher, Circle Limit II (1959)

M.C. Escher, Circle Limit IV (Heaven and Hell) (1960)

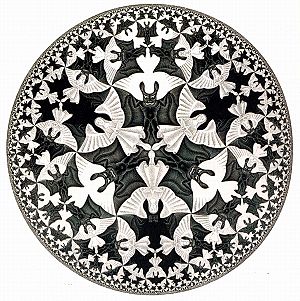
For Circle Limit II:

6. What is the highest order of rotation?
7. Draw geodesics in this figure. Describe the underlying geometric tessellation.

For Circle Limit IV:

8. What is the highest order of rotation? What other orders of rotation are present?
9. Draw geodesics in this figure. Describe the underlying geometric tessellation.
10. Draw a geodesic NOT passing through the center point.
11. How many geodesics can you draw through the center point, so that the new geodesic does not meet the geodesic you picked in the previous question? Another way to ask the same question: How many geodesics pass through the point so that the new geodesic is parallel to the first geodesic?

Circle Limit III

M.C. Escher, Circle Limit III, 1959.

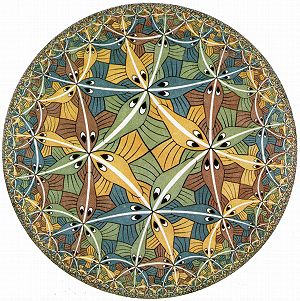
This Circle Limit is the most subtle. The white lines look like the geodesics in the other Circle Limit prints, but they are not the same. A closer look shows that these white lines are not geodesics at all.

12. Pick a triangle and determine its corner angles by considering the number of polygons at a vertex. Assume all angles at each vertex are equal (they are, though the distortion makes this harder to believe).
13. What is the sum of the angles in the triangle? Is this possible in hyperbolic geometry?
14. Choose a white line and trace it to the point where it meets the boundary of the disk. Carefully measure the angle it makes with the edge of the disk (you may want to draw tangent lines to the disk and the white line). What angle did you get?

Handin: Marked up Circle Limit prints and a sheet with answers to all questions.