More on Isometries Exploration

Objective:

What if we want to decorate a pillar or an inner-tube (called a torus by geometers)? We will develop some geometry that will allow us to create tessellations on these objects derived from Escher’s tessellations.

Column at a school in The Hague, the Netherlands

Isometries play an important role in the study of advanced topology and geometry. Remember that we have different types of isometries: translation, rotation, and reflection. Sometimes glide-reflections are mentioned as a fourth type of isometry. It is fairly easy to see that a glide-reflection is actually a combination of a reflection and a translation.

1. Draw a simple figure. Draw the image of your figure after some horizontal translation. Show that you can arrive at the same resulting image by performing two reflections. Show the lines of reflection in your diagram.
2. Draw a figure and its image after a diagonal translation. If you are allowed only reflections, how many do you need to arrive at the same image? Show the lines of reflection in your diagram.
3. Draw a figure and its image after a 45° rotation. If you are allowed only reflections, how many do you need to arrive at the same image? Show the lines of reflection in your diagram.

You might ask yourself at this point: if reflections describe all the other isometries, why do we bother to study the other isometries? The answer is that there are applications where we don’t want to think of for instance a rotation as a composition of two reflections. In certain settings we really need to understand the general theory of isometries. There will be some examples later on.

Tessellations and (groups of) isometries are closely related topics. If we choose our isometries carefully, then they actually generate a tessellation.

Tessellations by Isometries

Suppose we have two isometries, one called F which translates right by 1 unit, and one called G which translates up by 1 unit. An inverse isometry is an isometry that undoes whatever the original isometry did. So the inverse of F is to translate left by 1 unit, and the inverse of G is to translate down by 1 unit. The inverse isometries are written as $F^{-1}$ and $G^{-1}$ Start with a unit square, and apply the isometries F and G to the square several times. This results in a partial tessellation of the plane as shown below.

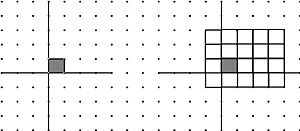
The unit square was carefully chosen so that applying the isometries would not cause any overlaps on its interior (just some on the edges). Such an area which tessellates the plane after the application of isometries is called a fundamental domain.

4. Draw a parallelogram with base of length one, and height of length one. One way to achieve this is with vertices at (0,0), (1,0), (1,1), and (2,1), but there are many other possibilites and you may use any. Apply the isometries F and G several times.
   1. What tessellation do you obtain? Provide a sketch.
   2. What is the fundamental domain?
5. Draw a unit square. The isometries are reflections in the sides of the square. Apply these isometries several times.
   1. What tessellation do you obtain? Provide a sketch.
   2. What is the fundamental domain?
   3. What is the inverse isometry of a reflection?
6. Draw an equilateral triangle. The isometries are reflections in the sides of the triangle. Apply this isometry several times.
   1. What tessellation do you obtain? Provide a sketch.
   2. What is the fundamental domain?
7. Draw a unit square with a right pointing arrow on the bottom and an upward pointing arrow on the left side. The isometries are translation by one unit in the horizontal direction, and a glide-reflection in the vertical direction. Apply this isometry several times.
   1. What tessellation do you obtain? Provide a sketch.
   2. What is the fundamental domain?
   3. What happens to the directions of the arrows after two glide-reflections?

Gluings

In some of the exercises we found similar tessellations and fundamental domains. To understand the real differences, we would need to look at how the isometries interact with the fundamental domain. We say that the isometries provide identifications for the sides of the fundamental domain. A translation provides a straightforward gluing, while a glide-translation provides a gluing with a twist. The reflections create a “border”. We will ignore the reflections for now.

8. Consider the fundamental domain from the example. We have a square with the left side glued to the right side, and the bottom glued to the top.
   1. If we take a square and glue to left side to the right side, what do you get?
   2. Now take this shape (from part a) and glue the top to the bottom. What do you get?
9. Consider the fundamental domain from question 4. We have a parallelogram with the left side glued to the right side, and the bottom glued to the top.
   1. If we take a parallelogram and glue to left side to the right side, what do you get?
   2. Now take this shape (from part a) and glue the top to the bottom. What do you get?
   3. Is there any difference from the resulting shape in question 8?
10. Consider the fundamental domain from question 8. We have a square with the left side glued to the right side, and the bottom glued to the top with a twist.
    1. If we take a square and glue to left side to the right side, what do you get?
    2. Now take this shape (from part a) and glue the top to the bottom with a twist. What do you get? (This space is called a Klein Bottle.)
    3. Can you do this last gluing in our regular 3-dimensional space? Explain.

Lattices

These isometries and fundamental domains are also apparent in Escher’s tessellations. Consider Sketch #47 (Birds). One way of finding the fundamental domain is to pick a point (choose a “vertex” type point) and looking for all copies of that point. (In mathematical terms we are looking for the orbit.) In this example take for instance the left wingtip of the white bird. If you use tracing paper, and mark all copies of this point you will get a grid of points (the technical term is a lattice). In this example you will see that the lattice traces out copies of a rhombus. The isometries that allow you to go from one side of the rhombus to the other are all translations. The fundamental domain is a rhombus decorated with 2 birds, the isometries are translations, hence the rhombus can be glued into a torus (donut-shape) nicely decorated with 2 birds!

11. 1. What happens if you take 2 copies of the fundamental domain before gluing the sides together?
    2. How many birds decorate this shape?
    3. What is the surface area compared to the original torus?
12. Consider Sketch #17 (Parrots).
    1. If you choose the tip of the beak and ignore color, what lattice do you get?
    2. What kind of fundamental domain do you get?
    3. If you pick two fundamental domains, where the two lie in a horizontal line, what shape do you get after gluing?
    4. If you pick two fundamental domains, where the two lie in a vertical line, what shape do you get after gluing?
13. Another variation on the same theme is to only glue some of the sides. Consider Sketch #27 (Butterflies and fish).
    1. Using the tip of the fish’s fin, what type of lattice do we get?
    2. What does the fundamental domain look like?
    3. Suppose we take a vertical strip of fundamental domains, and glue the left side to the right side. What shape do we get?
    4. Look at the column tiled with a flower pattern, Tiled Column, New Lyceum, Baarn. What does the tessellation of the plane look like from which this decoration is derived? Can you find the original tessellation of the plane?
    5. Consider Sketch #136 (Design drawing of tiles). How many different pillars can we construct from A1? Explain.

Handin: A sheet with answers to all questions.