Course:Hyperbolic Geometry Fall2008 - Bart

• Read Hyperbolic Geometry
• Write up solutions to the following questions:

1. Draw a Poincaré disk, and draw four geodesics that don't cross.
2. Draw a Poincaré disk, and draw four geodesics through the center point.
3. Copy the Poincaré disk shown below, and draw three geodesics through the point that don't cross the line shown.
   
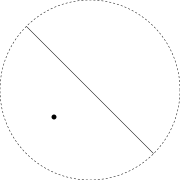
4. Draw a Poincaré disk, and draw a triangle with three 5° angles.
5. Draw a Poincaré disk, and draw a 90°-5°-5° triangle. (Hint: Put the 90° angle at the center point.)
6. Draw a Poincaré disk, and draw a pentagon with five right angles
7. Escher used angels and devils in three artworks: Sketch #45 (Angels and Devils), Sphere with Angels and Devils, and Circle Limit IV (Heaven and Hell).
   Answer each question for all three artworks:
   1. How many creatures touch at one wingtip?
   2. How many creatures touch at their feet?
   3. Each creature is basically a triangle formed by the two wingtips and the feet. From parts a & b, you know how many of these triangles come together at each vertex. What are the corner angles of these triangles?
   4. What are the angle sums of these triangles?
   5. What geometry is the artwork based on?
8. Which of these Escher artworks are based on hyperbolic geometry?
1. Path of Life I
2. Circle Limit I
3. Circle Limit II
4. Circle Limit III
5. Verbum
6. Development II
7. Square Limit
8. Snakes
9. Rippled Surface
9. Find the defect and area of the following hyperbolic polygons:
   1. A triangle with three 5° angles.
   2. A 90°-5°-5° triangle.
   3. A pentagon with five right angles.
   4. A 90°-0°-0° triangle. What does this triangle look like?
   5. A 20°-20°-20°-20° quadrilateral.
10. Compare Hyperbolic geometry to spherical and Euclidean geometry.
    • What can we say about parallel lines in hyperbolic geometry?
    • What can we say about the sum of the angles in a triangle?
    • Do n-gons exist for all possible values of n?
    • Do we have squares and rectangles in hyperbolic geometry? Why or why not?
    • Do parallellograms exist in hyperbolic geometry? If so, what do they look like?
    • Give an example (sketch) of the isometries: translation, rotation, and reflection.
    • How do we measure the defect of a triangle?
    • How do we measure the area of a triangle?
    • How do we measure the defect of a general polygon?
    • How do we measure the area of a general polygon?