Instructor:Hyperbolic Geometry Exercises Solutions

Hyperbolic Geometry Exercises

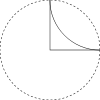
1. Four geodesics that don't cross.
2. Four geodesics through the center.
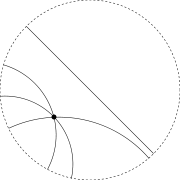
3. Three geodesics through point, parallel to given geodesic.
4. Triangle with three 5° angles.
5. 90°-5°-5° triangle.
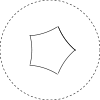
6. Right angled pentagon.
7. Fish in Circle Limit I alternate directions along geodesics, so that they are alternately head to head and tail to tail. In Circle Limit III, fish along the white lines all face the same direction, as if they could all be swimming forwards.
8. He's talking about Circle Limit III. He needed to print each of four colors plus black. With black, because of the fourfold rotation symmetry, he only carved a block to print ¼ of the circle. With the colors, he could have carved half circle blocks but didn't - he used two quarter-circle blocks for each color, each printing twice. So, (five colors) × (four impressions each) gives 20 impressions total.
9. • Sketch #45 (Angels and Devils): a) 8; b) 4; c) 45°-45°-90°; d) 180°; e) Euclidean
   • Sphere with Angels and Devils: a) 6; b) 4; c) 60°-60°-90°; d) 210°; e) Spherical
   • Circle Limit IV (Heaven and Hell): a) 8; b) 6; c) 45°-45°-60°; d) 150°; e) Hyperbolic
10. Only b, c, and d, the Circle Limit artworks.
11. 1. Defect 165°. Area ${\frac {11}{12}}\pi$.
    2. Defect 80°. Area ${\frac {4}{9}}\pi$.
    3. Defect 90°. Area ${\frac {\pi }{2}}$.
    4. Defect 90°. Area ${\frac {\pi }{2}}$. .
    5. Defect 280°. Area ${\frac {14}{9}}\pi$.
12. a) {3,10}; b) All are 36°; c) 72°; d) $2\pi /5$; e) Draw {10,3}.
13. a) {6,4}; b) All are 90°; c) 180°; d) $\pi$; e) Draw {4,6}.
14. The angle sum of the underlying 4-gon is π/6 + π/6 +π/4 + π/4 = 5π/6; so defect is 7π/6; now use area formula.
15. The angle sum of the underlying 3-gon is π/6 + π/6 + π/6 = π/2; so defect is 3π/2; now use area formula.
16. a) 180°; b)Largest area $\pi$, smallest area 0.
17. Largest area $2{\pi }$ , smallest area 0.
18. The dual tessellation will consist of hyperbolic 7-gons.
19. a) 6, 12, 18, 24, 30, 6n; b) 5, 5, 1, 0, 0, 0; c) 7, 21, 63, 189, 567, $7\cdot 3^{n-1}$
21. {5,4} has angle sum 450°, defect 90°. {4,5} has angle sum 288°, defect 72°. Area ratio is 72/90 = 4/5.
22. {3,7} has angle sum (3/7)360°, defect 180/7 (approx. 25.71°). {7,3} has angle sum 840°, defect 60°. Area ratio is 3/7.
23. {3,5} has angle sum 216°, defect 36°. {5,3} has angle sum 600°, defect 60°. Area ratio is 36/60 = 3/5.
24. Draw a picture with both tessellations in duality. Notice the triangles are made of three quadrilateral tiles, and the hexagons are made of six. So, the area ratio is 3/6 = 1/2
25. Area ratio is k/n (or n/k). For a proof, the argument in question 24 generalizes.