Hyperbolic Geometry Exercises

1. Draw a Poincaré disk, and draw four geodesics that don't cross.
2. Draw a Poincaré disk, and draw four geodesics through the center point.
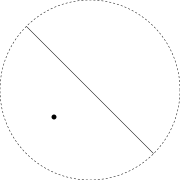
3. Copy the Poincaré disk shown below, and draw three geodesics through the point that don't cross the line shown.
   
4. Draw a Poincaré disk, and draw a triangle with three 5° angles.
5. Draw a Poincaré disk, and draw a 90°-5°-5° triangle. (Hint: Put the 90° angle at the center point.)
6. Draw a Poincaré disk, and draw a pentagon with five right angles
7. Escher wrote:

   This woodcut, Circle Limit I, being a first attempt, displays all sorts of shortcomings. Not only the shape of the fish, still hardly developed from rectilinear abstractions into rudimentary animals, but also their arrangement and relative position leave much to be desired...There is no continuity, no “traffic flow”, no unity of color in each row.

   Explain what Escher means by "traffic flow". Compare traffic flow in Circle Limit I and Circle Limit III.

8. Escher wrote, in a letter to his son Arthur:

   I've been killing myself, first to finally finish that litho and then, for four days with clenched teeth, to make another nine good prints of that highly painstaking circle-boundary-in-color. Each print requires twenty impressions: five blocks, each block printing four times.

   Which of the Circle Limit prints is Escher talking about? Explain the twenty impressions.

9. Escher used angels and devils in three artworks: Sketch #45 (Angels and Devils), Sphere with Angels and Devils, and Circle Limit IV (Heaven and Hell).
   Answer each question for all three artworks:
   1. How many creatures touch at one wingtip?
   2. How many creatures touch at (or near) their feet?
   3. Each creature is basically a triangle formed by the two wingtips and the feet. From parts a & b, you know how many of these triangles come together at each vertex (both wingtips and feet). What are the angles of these triangles? (Note both wingtip- and feet-angles.)
   4. What are the angle sums of these triangles?
   5. What geometry is the artwork based on: planar, spherical, or hyperbolic?
10. Which of these Escher artworks are based on hyperbolic geometry?
1. Path of Life I
2. Circle Limit I
3. Circle Limit II
4. Circle Limit III
5. Verbum
6. Development II (First version)
7. Square Limit
8. Snakes
9. Rippled Surface
11. Find the defect of the following hyperbolic polygons:
    1. A triangle with three 5° angles.
    2. A 90°-5°-5° triangle.
    3. A pentagon with five right angles.
    4. A 90°-0°-0° ideal triangle. What does this triangle look like?
    5. A 20°-20°-20°-20° quadrilateral.

12. 

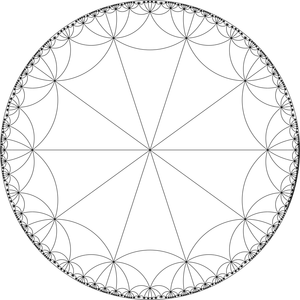
    Consider the hyperbolic tessellation by triangles shown to the right.

    1. What is its Schläfli symbol?
    2. What are the corner angles of the triangle?
    3. What is the defect of each triangle?
    4. What is the area of each triangle?
    5. Draw the dual tessellation. What is the Schläfli symbol of the dual tessellation?

13. 

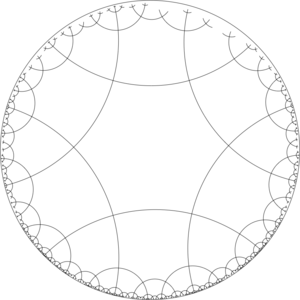
    Consider the hyperbolic tessellation by regular hexagons shown to the right.

    1. What is its Schläfli symbol?
    2. What are the corner angles of the hexagons?
    3. What is the defect of each hexagon?
    4. What is the area of each hexagon?
    5. Draw the dual tessellation. What is the Schläfli symbol of the dual tessellation?
14. What are the area(s) of the polygons in the underlying geometric tessellations of Circle Limit I?
15. What are the area(s) of the polygons in the underlying geometric tessellations of Circle Limit II?
16. 1. What is the upper limit for the defect of a hyperbolic triangle?
    2. What is the largest area a triangle can have? What is the smallest area a triangle can have?
17. What is the largest area a quadrilateral can have? What is the smallest area a quadrilateral can have?
18. Describe the dual of the hyperbolic tessellation by triangles shown below.

    

    What does the dual tessellation look like?

19. 1. Consider the tessellation of the plane with equilateral triangles. Pick a vertex. It has 6 neighbors (points which are 1 edge away). It has 12 “two-away neighbors”. Fill in the rest of the table:

       1-away neighbors	6
       2-away neighbors	12
       3-away neighbors
       4-away neighbors
       5-away neighbors
       n-away neighbors

    2. Now do the same for the icosahedron. Pick a vertex. It has 5 neighbors this time. Fill in the rest of the table.. be careful - you'll need to count them.

       1-away neighbors	5
       2-away neighbors
       3-away neighbors
       4-away neighbors
       5-away neighbors
       n-away neighbors

    3. Now do the same for the “hyperbolic paper” model of hyperbolic space (it might help to look at the $\{3,7\}$ hyperbolic tessellation). Pick a vertex. It has 7 neighbors this time. You can count the 2-away neighbors, but then things get hard. The formula for n-away neighbors is quite difficult.

       1-away neighbors	7
       2-away neighbors
       3-away neighbors
       4-away neighbors
       5-away neighbors
       n-away neighbors

20. Compare Hyperbolic geometry to spherical and Euclidean geometry.
    • What can we say about parallel lines in hyperbolic geometry?
    • What can we say about the sum of the angles in a triangle?
    • Do n-gons exist for all possible values of n?
    • Do we have squares and rectangles in hyperbolic geometry? Why or why not?
    • Do parallellograms exist in hyperbolic geometry? If so, what do they look like?
    • Give an example (sketch) of the isometries: translation, rotation, and reflection.
    • How do we measure the defect of a triangle?
    • How do we measure the area of a triangle?
    • How do we measure the defect of a general polygon?
    • How do we measure the area of a general polygon?
21. Consider the dual hyperbolic tessellations {5,4} and {4,5}. What is the defect of the tessellating polygon for each? What is the ratio of their areas?
22. Consider the dual hyperbolic tessellations {3,7} and {7,3}. What is the defect of the tessellating polygon for each? What is the ratio of their areas?
23. Consider the dual spherical tessellations {3,5} and {5,3}. What is the defect of the tessellating polygon for each? What is the ratio of their areas?
24. Sketch the dual Euclidean tessellations {6,3} and {3,6}. What is the ratio of the area of the hexagon to the area of the triangle?
25. Generalize the previous four exercises to give a formula for the ratio of the areas of a polygon from the ${n,k}$ tessellation and a polygon from the dual ${k,n}$ tessellation.

Instructor:Hyperbolic Geometry Exercises Solutions